During the late 19th and early 20th centuries, there was a problem that stumped even the best minds in physics. Eventually, one man, Max Planck, solved the problem, but his solution was one that was totally out of left field. While the math worked, he didn't actually believe the mathematics explained reality. It turned out his discovery was more true than he realized, and it ushered in a revolution in the world of physics that completely changed our view of nature and reality.
Learn more about the ultraviolet catastrophe and the birth of quantum mechanics on this episode of Everything Everywhere Daily. Ready to launch your business? Get started with the commerce platform made for entrepreneurs. Shopify is specially designed to help you start, run and grow your business with easy, customizable themes that let you build your brand. Marketing tools that get your products out there.
Integrated shipping solutions that actually save you time. From startups to scale-ups, online, in person, and on the go. Shopify is made for entrepreneurs like you. Sign up for your $1 a month trial at shopify.com. For those of you listening with your kids, I know it can be hard to find good content that you can listen to together. If you and your kids love a good, fun, and scary story, one that sends a tingle down your spine, I've got a great podcast recommendation for you.
R.L. Stine's story club and podcast from Go Kid Go. Ivy is the wicked cool gatekeeper to horror master R.L. Stine's secret story vault. The spooktacular tales she shares with R.L. Stine's Story Club members will give you chills and thrills galore. And if your kids love goosebumps, you'll die for the nonstop monsters, ghosts, and ghouls in R.L. Stine's Story Club.
So what are you waiting for? Listen to R.L. Stine Story Club today on Spotify, Apple, or wherever you get your podcasts. R.L. Stine and Ivy are waiting for you. This episode is one that I've been putting off doing for a very long time. That's because quantum mechanics is extremely complicated and counterintuitive, and as such, it is difficult to explain.
However, I always take solace in the words of the Nobel Prize-winning physicist Richard Feynman, who said, quote, I think I can safely say nobody understands quantum mechanics. The one element of quantum mechanics that probably can be easily understood is the story of exactly how it was developed and what problem it was initially trying to solve. It all started with a problem that plagued physics in the late 19th century.
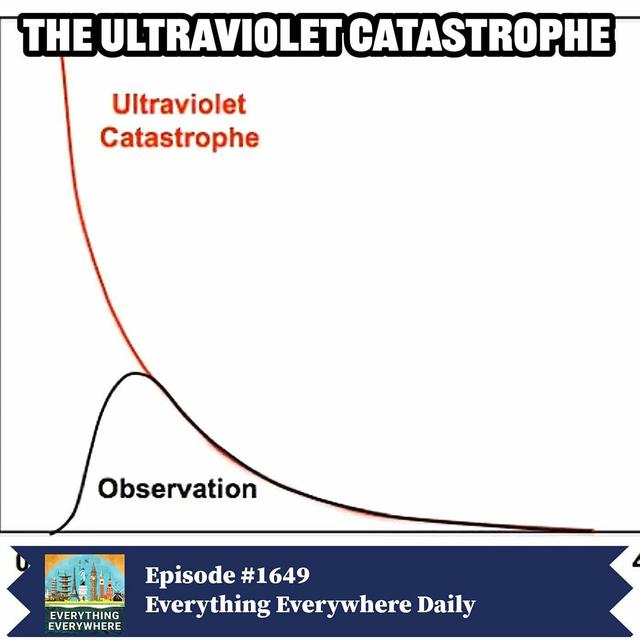
known as the ultraviolet catastrophe. To understand what the ultraviolet catastrophe was, we need to understand something called black body radiation. Blackbody radiation refers to the electromagnetic radiation emitted by an idealized object called a blackbody, which perfectly absorbs and admits all frequencies of radiation. A black body is a theoretical construct that reflects no light, meaning all electromagnetic radiation that lands upon it is absorbed. When heated,
a black body emits electromagnetic radiation in a spectrum that depends solely on its temperature, not its material composition. To measure black body radiation, experimental setups involve creating an approximate black body. a cavity with a small hole in its surface. This design ensures that any radiation entering the cavity would be absorbed and not reflected out, meaning that the hole behaves as a near-perfect blackbody emitter.
When the cavity was heated, the radiation escaping through that hole closely approximated true black body radiation. The cavity was often constructed with materials with high thermal conductivity, such as metals, to ensure uniform temperature distribution. Inside the cavity, the walls were coated with materials that absorbed nearly all incident radiation such as soot,
graphite, or other blackened substances. In the late 19th century, physicists sought to describe the spectrum of black body radiation using classical physics. The most prominent classical prediction came from the Rayleigh-Jean's Law, which described how radiation intensity varied with wavelength. According to the Rayleigh-Jean's law, the intensity of radiation would increase indefinitely as the wavelength decreased, leading to an infinite amount of energy being emitted at short wavelengths.
or the ultraviolet region of the spectrum. This result of infinite energy was physically impossible and thus became known as the ultraviolet catastrophe. The problem was that experimental results did not match the theory beyond a certain point. At lower wavelengths such as infrared radiation, the theory worked fine. Experimentally, it was observed that black body radiation did not behave as the Rayleigh-Jean's law predicted.
Instead, the intensity increased with decreasing wavelength only up to a certain point, after which it began to decline at shorter wavelengths. this discrepancy highlighted a major failure of classical physics to describe phenomena at high frequencies. The fact that the Rayleigh-Jean's law worked for some of the spectrum, but not all of the spectrum, frustrated physicists.
There was another law called Wien's Law, or the Viennese Law of Radiation, which was the opposite of the Rayleigh-Jean's Law. This law worked well at shorter wavelengths and higher frequencies, but failed to describe the spectrum at longer wavelengths and lower frequencies. When there's a problem between theory and reality, the theory has to change.
The ultraviolet catastrophe was one of the single biggest problems in the world of physics in the late 19th century. Nothing in conventional physics could explain why the black bodies behaved as they did. The solution to this dilemma came in the year 1900 from a 42 year old professor of physics at the University of Berlin, Max Planck. Planck approached the problem with a bold assumption. Rather than energy being continuous, as classical physics assumed, it might be discrete or quantized.
He proposed that energy could only be emitted or absorbed in discrete packets or quanta with the energy of each quantum proportional to its frequency. This became known as Planck's postulate. Here I want to explain the difference between continuous and discrete. I've touched on this in previous episodes, but the ideas are very easy to understand. The difference between continuous and discrete...
lies in the way values or elements are represented. Continuous refers to something that is unbroken or uninterrupted. If you draw a line on a piece of paper without lifting your pen, that would be continuous. In contrast, discrete refers to distinct or separate elements with values that are countable and not infinitely divisible. So if you drew a series of dots on a piece of paper with very small spaces in between,
that would be discrete and not continuous, even if it looks like a solid line from a distance. Another analogy is often used to explain the difference between continuous and discrete, and that is something continuous is like a slide. whereas something discrete are like steps. When you go down a slide, every point is lower than the one before it. With steps, however, you are on one step or another, and there's nothing in between.
Classical physics at this point assumed that energy was continuous. You could keep dividing it up indefinitely. For Planck's solution to work however, you had to assume that energy came from individual packets, also known as quanta, which came in discrete energy levels. This was a radical change in the world of physics, which had contended that light, aka electromagnetic radiation, came in the form of continuous waves. Or it would have been except for the fact that
Planck didn't actually think that it was true. Planck thought that his solution to the problem was nothing more than a mathematical workaround. He didn't actually think that energy came in quantized packets. It was just a trick to make the math work. Planck was deeply rooted in classical physics and found the idea of quantization philosophically troubling. In fact, he viewed this hypothesis as a provisional, somewhat artificial assumption rather than a reflection of the true nature of reality.
So having created a theory that fit the data, he then set about trying to resolve his theory to classical physics for the next several years. At a fundamental level, he simply couldn't believe that the world worked in the way his theory described. He explained in his own autobiography, quote,
My futile attempts to fit the quantum somehow into the classical theory continued for a number of years, and they cost me a great deal of effort. Many of my colleagues saw in this something bordering on a tragedy. But I feel differently about it. Now I knew the quantum played a far more significant part in physics than I had originally been inclined to suspect. End quote. End quote. End quote.
It wasn't until other physicists, particularly Albert Einstein, expanded on Planck's ideas that the full implications of quantization began to emerge. Einstein's 1905 work on the photoelectric effect demonstrated that light itself behaves as if it's composed of discrete packets of energy. The discovery of the photoelectric effect was the discovery that won Einstein a Nobel Prize.
not his work on relativity. So what is the photoelectric effect? The photoelectric effect is a phenomenon in which light shining on a material typically a metal, causes the ejection of electrons from that material surface. The effect was first observed in the late 19th century but could not be explained using classical physics. The photoelectric effect was another great unsolved problem of physics at the turn of the century.
Classical wave theory, which again treated light as a continuous wave, predicted that the energy of ejected electrons should increase with the intensity of light regardless of its frequency. However, experimental observations showed that the energy of the ejected electrons depended on the light's frequency, not its intensity.
In 1905, Albert Einstein provided a groundbreaking explanation that introduced the concept of light behaving as discrete packets of energy. The same solution that Planck used to solve the ultraviolet catastrophe problem. In fact, Einstein's solution used the Planck constant, the same constant that was used by Planck himself in his equation.
This was a bold departure from classical wave theory and suggested that light's quantization was not just a mathematical convenience, but a fundamental aspect of nature. Planck initially resisted Einstein's interpretation as he struggled to reconcile it with his classical worldview. Over time, however, as quantum theory developed and more experimental evidence was accumulated,
Planck came to accept that quantization was a fundamental principle. Even so, his initial reluctance underscores how revolutionary and counterintuitive the concept of quantization was at the time. And this wasn't the end of the use of quanta to solve physics problems. In fact, it was just the beginning. In 1913, the Danish physicist Niels Bohr developed a model of the hydrogen atom that incorporated quantum ideas.
He proposed that electrons orbit the nucleus in specific quantized orbits and could jump between these orbits by absorbing or emitting photons of specific energies. In 1924, the French physicist Louis de Broglie proposed that particles, such as electrons, exhibit wave-like properties. This idea was later confirmed by electron diffraction experiments establishing wave-particle duality.
The idea that particles like electrons could behave like waves, or that light could behave like a particle, once again made no intuitive sense. Yet that is exactly what the theories and the experimentation bore out. In 1926, Max Born provided the statistical interpretation of the wave function, suggesting that finding a particle in a particular state was based on probability. It couldn't be absolutely determined.
Planck wasn't the only physicist who doubted the very science he helped create. When Born's paper came out indicating that particles could only be determined probabilistically, Einstein wrote him a letter that said, quote, The theory produces a good deal but hardly brings us closer to the secret of the old one. I am at all events convinced that he does not play dice. End quote. And this quote has often been rephrased as simply,
While Einstein could accept quantized particles, his view of the world was deterministic. Cause followed effect. If you could go back and replay the universe, it would have to turn out the same way. Bourne's theory upended Einstein's worldview, and he couldn't believe it. And this wasn't even close to the end of it. Discoveries just kept getting weirder and weirder and at every step along the way, some physicists expressed disbelief at the findings.
In 1927, Werner Heisenberg introduced the uncertainty principle, which states that it is impossible to simultaneously know a particle's position and momentum with arbitrary precision.
The principle of superposition is a fundamental principle in quantum mechanics where a quantum system can exist in multiple states simultaneously until it is measured or observed. In 1935, physicist Erwin Schrödinger created a thought experiment to explain superposition whereby a cat would be both alive and dead inside of a box until it was observed. Once again, many physicists couldn't accept his theory because it made no intuitive sense.
Quantum entanglement is a phenomenon in which two or more particles become interconnected, such that the state of one particle is instantaneously correlated with the state of the other, regardless of the distance between them. And once again, Einstein was not comfortable with the implications of this, even though he was one of the men who helped develop the theory. He called it spooky action at a distance.
All of these various theories, which were later proven experimentally, make up the branch of science we know today as quantum mechanics. Some of the greatest physicists of the 20th century expressed disbelief at the very discoveries that they helped make. It's because the world we live in is very different than the world at the quantum level, even though the quantum world ultimately makes up our world.
It's like watching a big screen TV and seeing pictures and images, but when you put your face up close to the screen, you see nothing but tiny dots. To me, the ultimate lesson that can be derived from the works of Max Planck, Albert Einstein, and others is that more than trusting your instinct, you should always trust the math.
The executive producer of Everything Everywhere Daily is Charles Daniel. The associate producers are Benji Long and Cameron Kiever. I want to give a big shout out to everyone who supports the show over on Patreon, including the show's producers. Your support helps me put out a show every single day. And also, Patreon is currently the only place where Everything Everywhere Daily merchandise is available to the top tier of supporters.
If you'd like to talk to other listeners of the show and members of the Completionist Club, you can join the Everything Everywhere daily Facebook group or Discord server. Links to everything are in the show notes.